Introduction
The study of chaos theory has been a significant area of research in mathematics and physics, particularly in the context of nonlinear dynamical systems. Chen's system, proposed by Chinese mathematician Shangyou Chen in 1989, is a classic example of a chaotic system. This article aims to analyze the hyper-chaos generated from Chen's system, exploring its characteristics, generation mechanisms, and implications in various fields.
Background and Definition of Hyper-Chaos
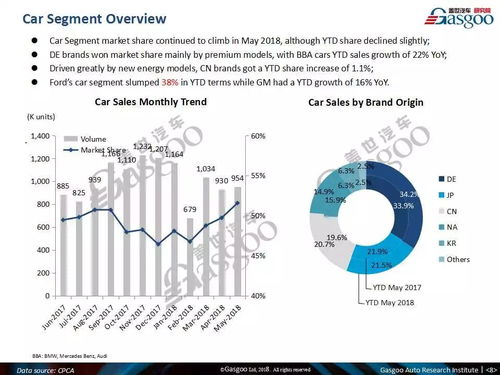
Chen's system is a three-dimensional autonomous dynamical system defined by the following equations:
[ begin{align}
x' &= alpha x - yz,
y' &= xz - beta y,
z' &= xy - gamma z,
end{align} ]
where ( alpha, beta, gamma ) are system parameters. The system exhibits chaotic behavior for certain parameter values, leading to the generation of hyper-chaos, which is a higher-dimensional chaotic attractor.
Hyper-chaos is a term used to describe chaotic behavior in systems with more than three dimensions. It is characterized by the presence of at least one positive Lyapunov exponent, indicating exponential growth of small perturbations, and the presence of a complex attractor with a fractal structure.
Characteristics of Hyper-Chaos in Chen's System

The hyper-chaos in Chen's system can be analyzed through various methods, including phase portraits, Lyapunov exponents, and bifurcation diagrams. Here are some key characteristics:
1. Phase Portraits: Phase portraits of Chen's system with hyper-chaotic behavior show complex attractors with a fractal structure. These attractors are typically non-symmetric and have a high degree of sensitivity to initial conditions.

2. Lyapunov Exponents: The Lyapunov exponents provide a quantitative measure of the chaotic behavior. In the case of Chen's system, the presence of at least one positive Lyapunov exponent indicates the hyper-chaotic nature of the system.

3. Bifurcation Diagrams: Bifurcation diagrams reveal the changes in the system's behavior as the parameters are varied. For Chen's system, the bifurcation diagram shows a transition from regular to chaotic and eventually to hyper-chaotic behavior as the parameters cross certain thresholds.

Generation Mechanisms of Hyper-Chaos

The generation of hyper-chaos in Chen's system can be attributed to several factors:
1. Parameter Sensitivity: The system is highly sensitive to changes in the parameters ( alpha, beta, gamma ). Even small variations in these parameters can lead to significant changes in the system's behavior, contributing to the hyper-chaotic dynamics.

2. Nonlinearity: The nonlinear terms in the equations of Chen's system play a crucial role in generating chaos. The interactions between these nonlinear terms lead to the complex dynamics observed in the system.

3. Feedback Loops: The feedback loops inherent in the system's equations contribute to the amplification of small perturbations, leading to the exponential growth of chaos.

Applications and Implications

The hyper-chaotic behavior of Chen's system has implications in various fields, including physics, engineering, and biology. Some of the applications include:
2. Engineering: The chaotic behavior can be utilized in secure communication systems, where the sensitivity to initial conditions can be exploited to create unpredictable and secure channels.

Conclusion

In conclusion, the analysis of hyper-chaos generated from Chen's system reveals a rich and complex dynamical behavior. The system's sensitivity to parameters, nonlinearity, and feedback loops contribute to the generation of hyper-chaotic attractors. The implications of this behavior in various fields highlight the importance of understanding and harnessing chaos for practical applications. Further research into the properties and applications of hyper-chaos in Chen's system and other chaotic systems is essential for advancing our knowledge of complex dynamical systems.
